Skip to main content

Section 4.2 The proof that the minimizing arc is a catenary

As we have already noted in Section 1.3, the integral which we shall have to minimize for the problem of determining a surface of revolution of minimum area is

\begin{equation*} I=\int_{x_1}^{x_2} f(y, y')\,dx \end{equation*}

where \(f(y, y')\) now has the value

\begin{equation} f(y, y') = y\sqrt{1+(y')^2}.\label{eqn-4-2}\tag{4.2.1} \end{equation}

The curves to be studied for this problem must all lie in the upper half-plane \(y\geq 0\) since on an arc with portions below the \(x\)-axis the value of the integral \(I\) is the difference of the areas generated by the segments above and the segments below the axis, while we are wishing to consider the sum of those areas. If an arc has segments below the \(x\)-axis it may always be replaced by one above the axis which will generate the same surface, as is clear from Figure 4.2.1. Besides the restriction \(y\geq 0\) our curves \(y=y(x)\) we shall, as in the two preceding examples, assume that all the curves \(y=y(x)\) considered are continuous and have tangents which turn continuously except possibly at a finite number of corners. Let us call curves of this sort in the upper half-plane admissible curves.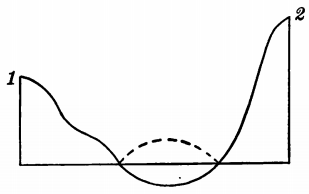

Any portion of the curve below the x-axis may be reflected over the x-axis without changing the area of the surface of revolution.
Figure 4.2.1.

Our problem is then to determine among all admissible arcs joining two given points 1 and 2 one which minimizes the integral \(I\text{.}\)

The necessary conditions deduced in Section 3.3 apply without alteration to our present problem. The minimizing arcs must be solutions of the equation

\begin{equation*} f_{y^{\prime}}=\int_{x_{1}}^{x} f_{y}\,dx+c \end{equation*}

which for the special function (4.2.1) takes the form

\begin{equation*} \frac{y\cdot y^{\prime}}{\sqrt{1+(y')^2}}=\int_{x_{i}}^{x} \sqrt{1+(y')^2}\,dx+c=s+c \end{equation*}

where \(s\) is the length of the minimizing arc measured from 1 to the point whose abscissa is \(x\text{.}\) At a point of the arc where \(y>0\) this equation can be solved for \(y'\text{,}\) giving

\begin{equation} y^{\prime}=\frac{s+c}{\sqrt{y^{2}-(s+c)^{2}}}\label{eqn-4-3}\tag{4.2.2} \end{equation}

and we see at once that at such a point \(y'\) is continuous since \(y\) and \(s\) both have this property. But if \(y'\) is continuous then \(y\) and \(s\) both have continuous derivatives and the equation (4.2.2) shows again that \(y'\) must also have a continuous derivative At all points above the \(x\)-axis our minimizing arc has therefore continuous curvature and no corners.

If we know that along a minimizing arc there is a continuous derivative \(y''\) then as in Section 3.3 Euler's equation has the consequence \(f-y' f_{y'} = \textrm{constant,}\)

which for the special function (4.2.1) takes the form

\begin{equation} \frac{y}{\sqrt{1+(y')^2}} = b.\label{eqn-4-4}\tag{4.2.3} \end{equation}
By solving this equation for \(y'\) and integrating we see that the solutions of Euler's equation also satisfy the two equations
\begin{equation*} \frac{d y}{\sqrt{\left(\frac{y}{b}\right)^{2}-1}}=d x, \quad b \log \left(\frac{y}{b}+\sqrt{\left(\frac{y}{b}\right)^{2}-1}\right)=x-a, \end{equation*}
and it follows readily by solving the last one for \(y\) that the extremals for our problem are the catenaries
\begin{equation} y = \frac{b}{2} \left[\exp\left(\frac{x-a}{b}\right) + \exp\left(-\frac{x-a}{b}\right)\right] = b \cosh \frac{x-a}{b}.\label{eqn-4-5}\tag{4.2.4} \end{equation}
We use here and in the following pages the customary symbols \(\cosh u, \sinh u\) for the hyperbolic cosine and the hyperbolic sine of \(u\) defined by the equations
\begin{equation*} \cosh u = \frac{e^u + e^{-u}}{2}, \quad \sinh u = \frac{e^u - e^{-u}}{2}. \end{equation*}
No elaborate properties of these functions will be needed, but it will be helpful later if we notice, while these formulas are before us, that each of them has the other as its derivative.
Remark 4.2.2.

Here I've made a deliberate break from the book's notation. In the original text, these two functions are denoted \(\operatorname{ch} u\) and \(\operatorname{sh} u\text{.}\) While these names are still occasionally found in modern literature, I find them to be really old-fashioned, so I've replaced them throughout with the much more common \(\cosh u\) and \(\sinh u\text{.}\)

When we know that the extremals are the catenaries of Figure 4.1.2, we see at once that a minimizing arc \(y=y(x)\) with corners is impossible since the corners would have to be on the \(x\)-axis, as has already been indicated, and the parts of the minimizing arc above the axis would have to be segments of catenaries which have no points in common with the axis. We have justified, therefore, the following conclusion: