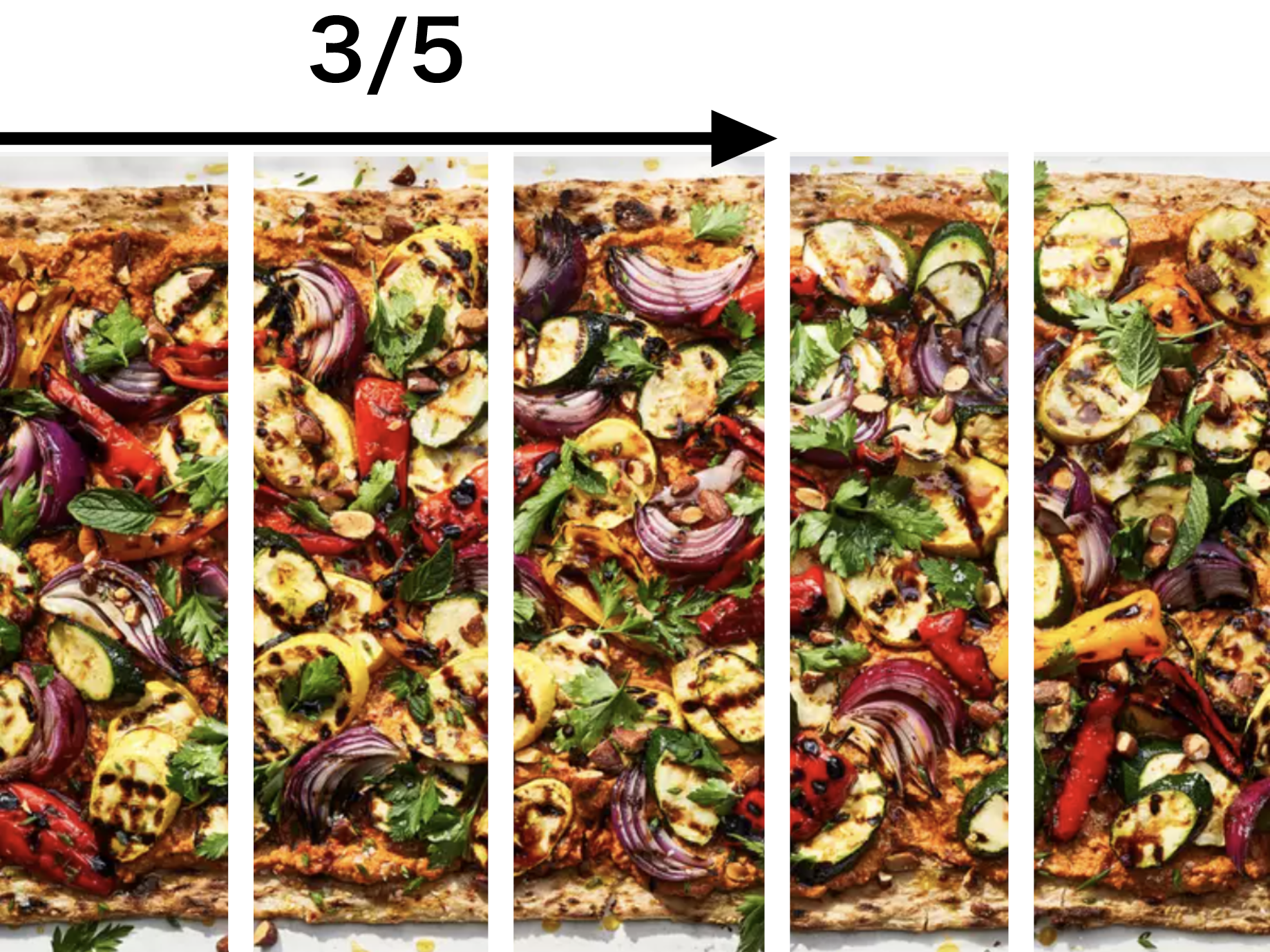
Piadina’s Restaurant specializes in flatbreads. My favorite is the grilled vegetable flatbread with smoked almond muhammara (a sauce made from almonds, red bell peppers, red chili peppers, pomegranate molasses, cumin, lemon juice, and olive oil). Last night I ordered that flatbread and a salad for dinner. They serve each flatbread cut into 5 equal slices.
Turns out the salad was pretty big and so I only ate 3 slices of flatbread. One way to describe how much of the flatbread I ate is using the fraction
\(\frac{3}{5}\text{.}\)
When I mentioned my dinner to my friend Hayfa she had a strange response: “Last time I ate a flatbread at Piadina’s I only ate \(\frac{2}{3}\) of what you ate.” I quickly figured it out that since I ate 3 slices and she ate \(\frac{2}{3}\) of that, she must have eaten 2 slices, which we can calculate as
\begin{equation*}
\frac{2}{3} \times 3 = 2 \div 3 \times 3 = 2
\end{equation*}
I started to obsesses over these fractions. Remember that I ate \(\frac{3}{5}\) of the flatbread and Hayfa ate \(\frac{2}{3}\) of what I ate. So she must have eaten \(\frac{2}{3}\) of \(\frac{3}{5}\text{,}\) right? That means
\begin{equation*}
\frac{2}{3} \times \frac{3}{5} = \frac{2}{5}
\end{equation*}
Ah yes! She ate 2 out of 5 slices, or \(\frac{2}{5}\) of the flatbread. That’s correct.
You might remember that we multiply fractions by multiplying the numerator (top) and the denominator (bottom). Let’s do that
\begin{equation*}
\frac{2}{3} \times \frac{3}{5} = \frac{2 \times 3}{3 \times 5} = \frac{6}{15}
\end{equation*}
Uh oh. We got a different answer.
Wait a minute. Check it out:
\begin{equation*}
\frac{2}{5} = 2 \div 5 = 0.4 \quad \text{ and } \quad \frac{6}{15} = 6 \div 15 = 0.4
\end{equation*}
Whew! The fractions \(\frac{2}{5}\) and \(\frac{6}{15}\) are equal.
To see why, consider what happens when I cut the flatbread the other direction twice. Now the entire flatbread has 15 squares. Hayfa ate 2 slices out of the original 5 slices total. Or, equivalently, she ate 6 squares out of 15 original squares. You may have heard of reducing or cancelling fractions, which is a way to remember what’s happening here:
\begin{equation*}
\frac{2 \times 3}{3 \times 5} = \frac{2 \times \cancel{~3~}}{ \cancel{~3~} \times 5} = \frac{2}{5}
\end{equation*}
In practice when we have to evaluate a product of fractions we can do it a couple of ways. One way is to deal with each fraction separately:
\begin{equation*}
\frac{2}{3} \times \frac{3}{5} = 2 \div 3 \times 3 \div 5 = 0.4
\end{equation*}
Another way is to calculate the top and bottom of the fraction and divide. Notice how we need to use parentheses around the top and bottom of the fraction.
\begin{equation*}
\frac{2}{3} \times \frac{3}{5} = \frac{2 \times 3}{3 \times 5} = (2 \times 3)\div (3 \times 5) = 0.4
\end{equation*}
By the way, flatbreads at Piadina’s are pricey: $17.49 each. At that rate, what does each slice cost? We can use a fraction
\begin{equation*}
\frac{\$17.49}{5 \text{ slices}} = \$17.49 \div 5 = 3.498 \approx \$3.50 \text{ per slice}
\end{equation*}
Notice that the units on my answer are dollars per slice: the units of the numerator (top) per the units of the denominator (bottom). We sometimes even write the units themselves as a fraction:
\begin{equation*}
\frac{\$}{\text{slice}}
\end{equation*}
There will be a few times during this course where you encounter fractions. Mostly we’ll switch to decimal approximations right away.